Wattmeters Polyphase 0 0 1.42 1.42 0 1.0 Wattmeters 5-ampere, 60-cycle Current Circuit AB-14, -15, Single- 0.063 0.019 0-00016 1.58 0.48 1.51 0.30 -16, -18, and -19, -30, Polyphase -40 Wattmeters TABLE II EXTERNAL RESISTORS Description Volts External Resistor Single-phase 100-125 None. EE 12 - Polyphase - Free download as Powerpoint Presentation (.ppt), PDF File (.pdf), Text File (.txt) or view presentation slides online.
Problems
1.The input power to a 3-phase a.c. motor is measured as 5kW. If the voltage and current to the motor are 400V and 8.6A respectively, determine the power factor of the system?
Power P=5000W,
line voltage VL = 400 V,
line current, IL = 8.6A and
power, P =√3 VLIL cos φ
Hence
power factor = cosφ=P√3VLIL
=5000 √3 (400) (8.6)
=0.839
2.Two wattmeters are connected to measure the input power to a balanced 3-phase load by the two-wattmeter method. If the instrument readings are 8kW and 4kW, determine (a) the total power input and (b) the load power factor.
(a)Total input power,
P=P1 +P2 =8+4=12kW

(b) tan φ =√3(P1 − P2)/(P1 + P2)
=√3 (8 – 4) / (8 + 4)
=√3 (4/12)
=√3(1/3)
Polyphase Wattmeter Pdf Online
= 1/ √3
Hence φ= tan−1 1 √3 =30◦
Power factor= cos φ= cos 30◦ =0.866
3.Two wattmeters connected to a 3-phase motor indicate the total power input to be 12kW. The power factor is 0.6. Determine the readings of each wattmeter.
If the two wattmeters indicate P1 and P2 respectively
Then P1 + P2 = 12kW | ---(1) |
tan φ =√3(P1 − P2)/(P1 + P2)
And power factor=0.6= cos φ.
Angle φ= cos−10.6=53.13◦ and
tan 53.13◦ =1.3333.
Hence
1.3333 =√3(P1 − P2)/12
From which,
P1 − P2 = 12(1.3333) /√3
i.e. P1 −P2 =9.237kW ----(2)
Adding Equations (1) and (2) gives:
2P1 = 21.237
i.e P1 = 21.237/2
= 10.62kW Hence wattmeter 1 reads 10.62kW From Equation (1), wattmeter 2 reads
Polyphase Wattmeter Pdf Download
(12−10.62)=1.38kW

4.Three loads, each of resistance 30, are connected in star to a 415 V, 3-phase supply. Determine
(a) the system phase voltage, (b) the phase current and (c) the line current.
A ‘415 V, 3-phase supply’ means that 415 V is the line voltage, VL
Polyphase Wattmeter Pdf Free
(a) For a star connection, VL =√3Vp Hence phase voltage, Vp = VL/√3
=415 /√3
=239.6 V or 240 V
correct to 3 significant figures
(b) Phase current, Ip = Vp/Rp
=240/30
=8 A
(c)For a star connection, Ip = IL Hence the line current, IL = 8 A
5.Three identical coils, each of resistance 10ohm and inductance 42mH are connected (a) in star and (b) in delta to a 415V, 50 Hz, 3-phase supply. Determine the total power dissipated in each case.
(a) Star connection

Inductive reactance,
XL =2πf L =2π (50) (42×10−3) =13.19
Phase impedance,
Zp =√(R2+XL2)
=√(102 +13.192) =16.55
Polyphase Wattmeter Pdf File
Line voltage, VL =415 V
And phase voltage,
VP =VL/√3=415/√3=240 V.
Phase current,
Ip =Vp/Zp =240/16.55=14.50 A. Line current,
IL =Ip =14.50 A.
Power factor= cos φ=Rp/Zp =10/16.55 =0.6042 lagging.
Power dissipated,
P =√3VLIL cosφ=√3 (415) (14.50)(0.6042) =6.3kW(Alternatively,
P =3I2R=3(14.50)2(10)=6.3kW)
(b) Delta connection
VL = Vp = 415 V,
Zp = 16.55_, cos φ = 0.6042lagging (from above). Phase current,
Ip =Vp/Zp =415/16.55=25.08A. Line current,
IL =√3Ip =√3(25.08)=43.44A.
Power dissipated,
P =√3VLIL cosφ
=√3 (415)(43.44)(0.6042) = 18.87kW
(Alternatively,
P =3I2R
=3(25.08)2(10) =18.87 kW)
6.A 415V, 3-phase a.c. motor has a power output of 12.75kW and operates at a power factor of 0.77 lagging and with an efficiency of 85 per cent. If the motor is delta-connected, determine (a) the power input, (b) the line current and (c) the phase current.
(a) Efficiency=power output/power input.
Hence
(85/100)=12.750 power input from which, Power input = 12. 750 × 10085
=15 000W or 15Kw
(b)Power, P=√3 VLIL cos φ, hence
(c)line current,
IL = P/ √3 (415)(0.77)
=15 000/ √3 (415) (0.77)
=27.10A
(d)For a delta connection, IL =√3 Ip,
Hence
Phase current,Ip = IL/√3
=27.10 /√3
=15.65A
7.A 400V, 3-phase star connected alternator supplies a delta-connected load, each phase of which has a resistance of 30_ and inductive reactance 40_. Calculate (a) the current supplied by the alternator and (b) the output power and the kVA of the alternator, neglecting losses in the line between the alternator and load.
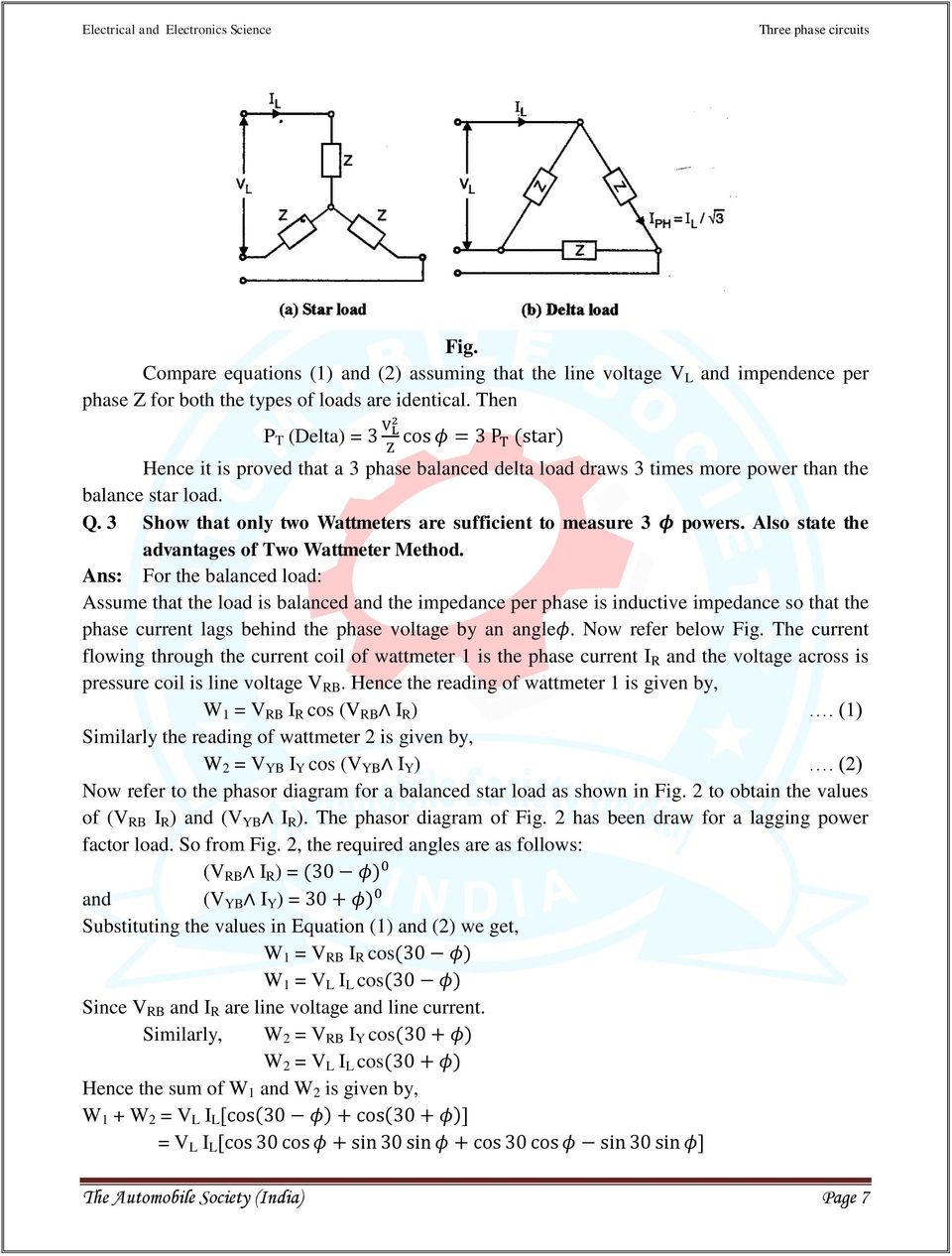
A circuit diagram of the alternator and load is shown in Fig.
(a) Considering the load:
Phase current, Ip =Vp/Zp
Vp =VL for a delta connection,
Hence Vp =400V. Wwf raw pc game free download.
Phase impedance,
Zp =√ (R2+XL2)
=√ (302 +402) =50
Figure
Hence Ip =Vp/Zp =400/50=8A.
For a delta-connection,
Line current, IL =√3 Ip =√3 (8) =13.86 A.
Hence 13.86A is the current supplied by the alternator.
(b) Alternator output power is equal to the power Dissipated by the load
I.e. P =√3 VLIL cos φ, Where cos φ = Rp/Zp = 30/50 = 0.6.
Hence P =√3 (400) (13.86) (0.6) = 5.76kW.

Alternator output kVA,
S=√3 VLIL =√3 (400) (13.86)
9.60 kVA.